
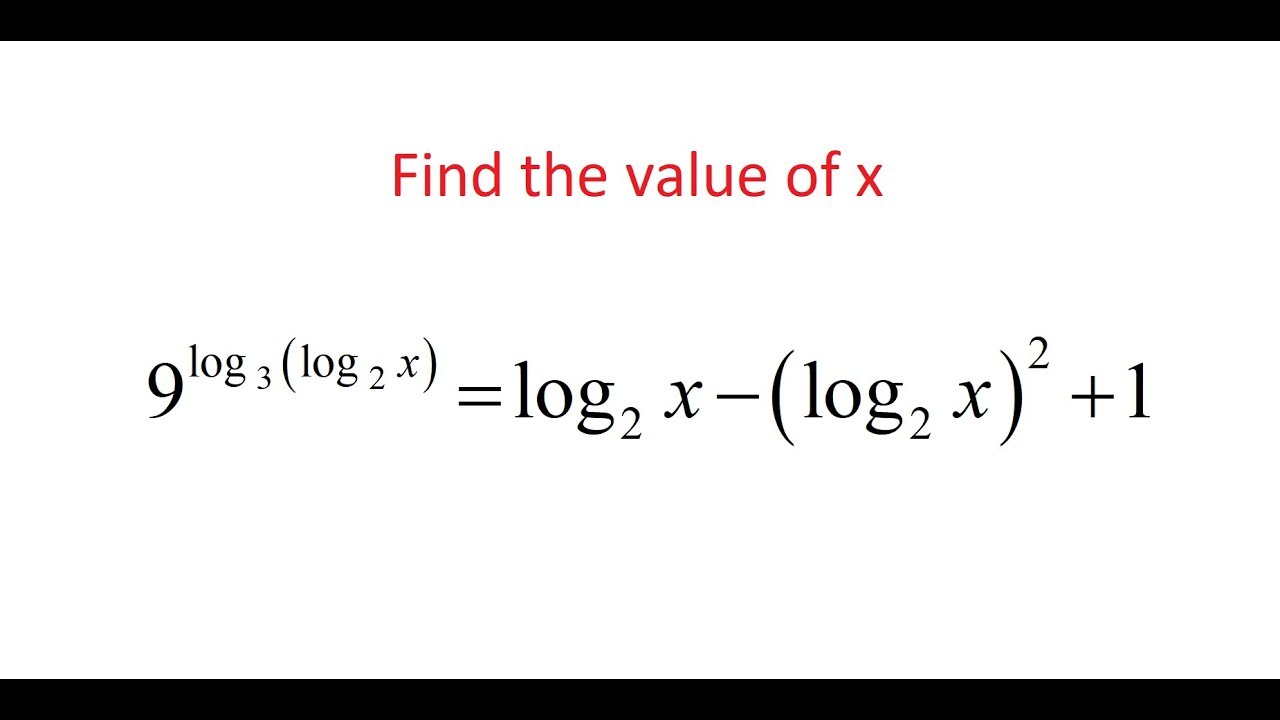
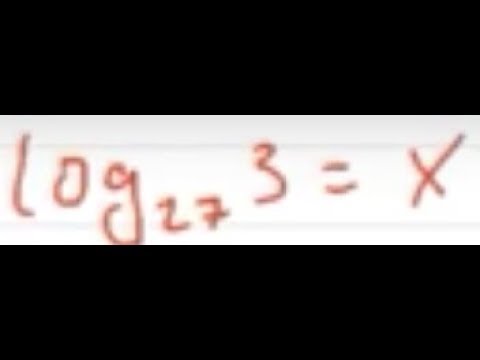
Two common variables involve natural logarithm: the GDP growth rate and the price elasticity of demand. Since growth rates often follow a similar pattern as the above example, economics also heavily rely on natural logarithms. Instead, it becomes somewhat stable: it's approaching a unique value already mentioned above, e ≈ 2.718281. You may notice that even though the frequency of compounding reaches an unusually high number, the value of (1 + r/m)ᵐ (which is the multiplier of your initial deposit) doesn't increase very much. Now let's check how the growing frequency affects your initial money: Now, let's imagine that your money is recalculated every minute or second: the m became a considerably high number. It is easy to see how quickly the value of m is increasing if you compare yearly (m=1), monthly (m=12), daily (m=365), or hourly (m=8,760) frequencies. Let's assume that you deposit some money for a year in a bank where compounding frequently occurs, thus m equal to a large number.

The formula for annual compound interest is as follows: That is the interest that is calculated on both the principal and the accumulated interest. One practical way to understand the function of the natural logarithm is to put in the context of compound interest. Therefore, y = logₑx = ln(x) which is equivalent to x = eʸ = exp(y). You might also see log(x), which also refers to the same function, especially in finance and economics. Accordingly, the logarithm can be represented as logₑx, but traditionally it is denoted with the symbol ln(x). Conventionally this number is symbolized by e, named after Leonard Euler, who defined its value in 1731. If you want to compute a number's natural logarithm, you need to choose a base that is approximately equal to 2.718281. Hence, the log2 value for different numbers can be calculated accordingly.Ĭalculate binary logarithm (log2) online using Log Base 2 Calculator.You can choose various numbers as the base for logarithms however, two particular bases are used so often that mathematicians have given unique names to them, the natural logarithm and the common logarithm. That is, the number of times, the number "2" should be multiplied by itself to obtain n.Īssume that we are required to find the log base 2 for the numbers 8, 32, 64. Hence, log2 calculation can be done using the below formula Log base 2 of a number "n" is the power to which the number "2" (base value) must be raised to obtain the value n.
LOG BASE 2 OF 6 HOW TO
Use this step by step tutorial to learn how to calculate log base 2. Here is a simple online tutorial that helps you to learn the basics of binary logarithm and log2 calculation. Generally, logarithm uses a base number to obtain a resultant number. The binary logarithm is generally denoted as lb(x) or log 2(x). This logarithm is used in bioinformatics, computer science, photography, maths, physics, chemistry and in other fields. In other words, log base 2 or the binary logarithm is how many times the number "2" is multiplied by itself to obtain the given value. That is Binary Logarithm (2) is multiplied by itself 3 times to obtain 8 and multiplied 5 times to obtain 32. For example, the binary logarithm of 1 is 0, 2 is 1, 8 is 3 and 32 is 5. The log2 of a number n is the power to which the number 2 must be raised to obtain the value n. The log base 2, also known as the binary logarithm uses the number 2 as the base. How to find the log base 2 (log2) value? Log2 Calculation


 0 kommentar(er)
0 kommentar(er)
